sextarius
is
disputed,
and
a
capacity
as
high
as
0-562
1.
or
0-99
imperial
pint
is
given
for
the
sextarius
by
an
actually
extant
measure.
This
would
give
as
the
capacity
of
the
ephah-bath
40-46
1.
or
71-28
pints.
But
it
is
highly
improbable
that
the
equation
of
log
to
sextarius
was
more
than
approximate.
It
is
more
easy
to
confound
closely
resembling
measures
of
capacity
than
of
length,
area,
or
weight.
Other
methods
of
ascertaining
the
capacity
of
the
ephah
are
the
following.
We
may
assume
that
it
was
the
same
as
the
Babylonian
unit
of
0
505
1.
(0-89
pint)
.
This
would
give
an
ephah
of
36-37
1.,
ornearly
8
gallons
or
66-5
sextarii
of
the
usually
assmned
weight,
and
more
or
less
f.quares
with
Epiphanius'
equation
oftheseahori
ephah
with
22
sextarii.
Or
we
may
connect
it
with
the
Egyptian
system,
thus:
both
the
ephah-bath
and
the
Egyptian
-Ptolemaic
artabe
are
equated
to
the
Attic
metrites
of
72
sextarii.
Now,
in
the
case
of
the
artabe
this
is
only
an
approximation,
tor
it
is
known
from
native
Egyptian
sources
(which
give
the
capacity
in
terms
of
a
volume
of
water
of
a
certain
weight)
that
the
artabe
was
about
36*45
1.,
or
a
little
more
than
64
pints.
Other
calculations,
as
from
a
passage
of
Josephus,
where
the
cor
is
equated
to
41
Attic
(Graeco-Roman)
modii
(i.e.
656
sextarii),
give
the
same
result.
In
this
passage
modii
is
an
almost
certain
emendation
of
medimni,
the
confusion
between
the
two
being
natural
in
a
Greek
MS.
There
are
plenty
of
other
vague
approximations,
ranging
from
60
to
72
sextarii.
Though
the
passage
of
Josephus
is
not
quite
certain
in
its
text,
we
may
accept
it
as
na-ving
the
appearance
of
precise
determination,
especially
since
it
gives
a
result
not
materially
differing
from
other
sources
of
information.
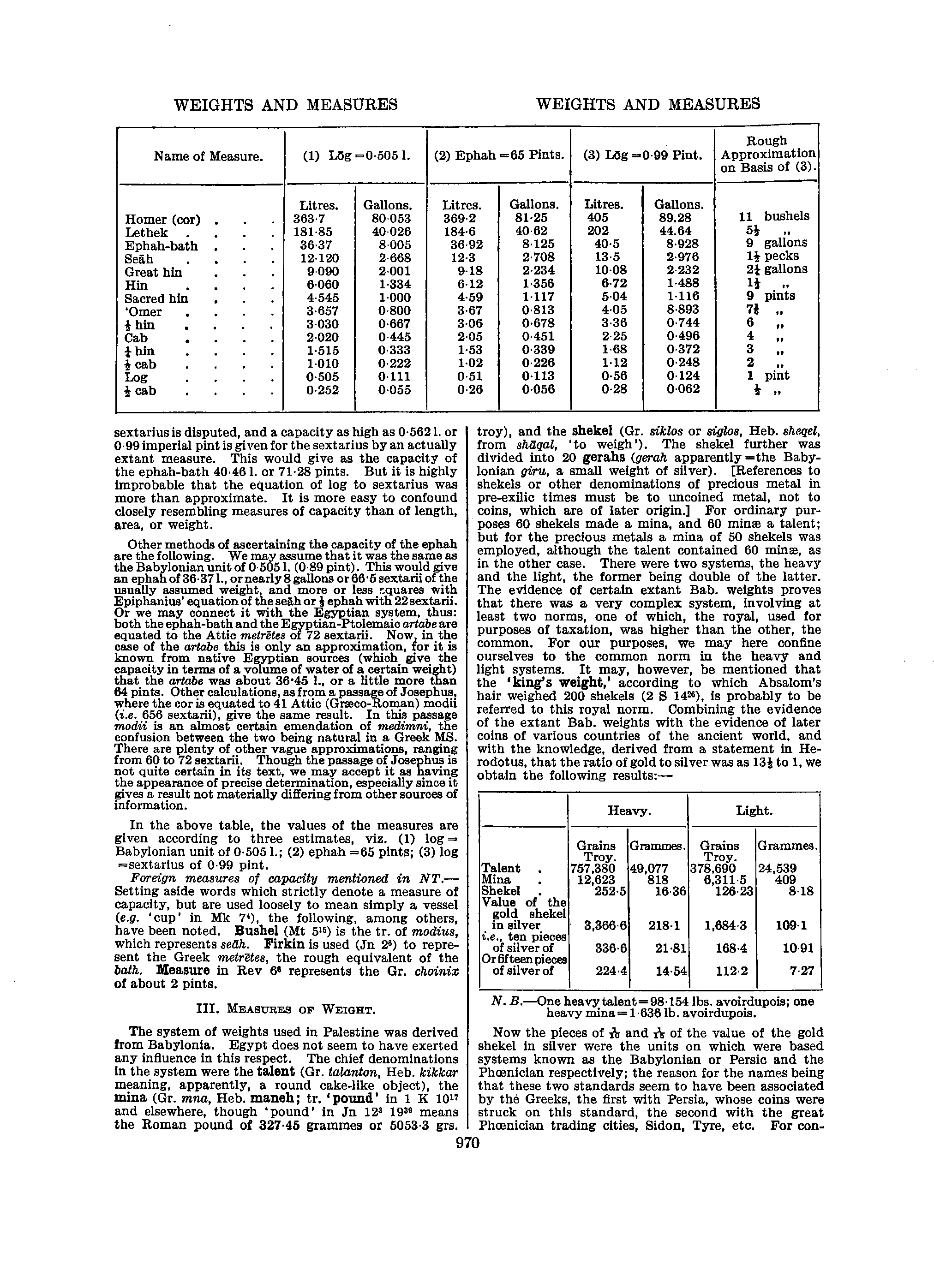
In
the
above
table,
the
values
of
the
measures
are
given
according
to
three
estimates,
viz.
(1)
log
=
Babylonian
unit
of
0-505
1.;
(2)
ephah
=65
pints;
(3)
log
=sextarius
of
0-99
pint.
Foreign
measures
of
capacity
mentioned
in
NT.
—
Setting
aside
words
which
strictly
denote
a
measure
of
capacity,
but
are
used
loosely
to
mean
simply
a
vessel
(e.g.
'cup'
in
Mk
7*),
the
following,
among
others,
have
been
noted.
Bushel
(Mt
S")
is
the
tr.
of
modius,
which
represents
seah.
Firkin
is
used
(Jn
2°)
to
repre-sent
the
Greek
metrites,
the
rough
equivalent
of
the
bath.
Measure
in
Rev
6'
represents
the
Gr.
choinix
of
about
2
pints.
III.
Measures
op
Weight.
The
system
of
weights
used
in
Palestine
was
derived
from
Babylonia.
Egypt
does
not
seem
to
have
exerted
any
influence
in
this
respect.
The
chief
denominations
in
the
system
were
the
talent
(Gr.
talanton,
Heb.
kikkar
meaning,
apparently,
a
round
cake-like
object),
the
mina
(Gr.
mna,
Heb.
maneh;
tr.
'pound'
in
1
K
10"
and
elsewhere,
though
'pound'
in
Jn
12'
19''
means
the
Roman
pound
of
327-45
grammes
or
5053-3
grs.
troy),
and
the
shekel
(Gr.
siklos
or
siglos,
Heb.
sheqel,
from
shaqal,
'to
weigh').
The
shekel
further
was
divided
into
20
gerahs
(gerah
apparently
—the
Baby-lonian
giru,
a
small
weight
of
silver).
[References
to
shekels
or
other
denominations
of
precious
metal
in
pre-exilic
times
must
be
to
uncoined
metal,
not
to
coins,
which
are
of
later
origin.]
For
ordinary
pur-poses
60
shekels
made
a
mina,
and
60
minae
a
talent;
but
for
the
precious
metals
a
mina
of
50
shekels
was
employed,
although
the
talent
contained
60
mins,
as
in
the
other
case.
There
were
two
systems,
the
heavy
and
the
light,
the
former
being
double
of
the
latter.
The
evidence
of
certain
extant
Bab.
weights
proves
that
there
was
a
very
complex
system,
involving
at
least
two
norms,
one
of
which,
the
royal,
used
for
purposes
of
taxation,
was
higher
than
the
other,
the
common.
For
our
purposes,
we
may
here
confine
ourselves
to
the
common
norm
in
the
heavy
and
light
systems.
It
may,
however,
be
mentioned
that
the
'king's
weight,'
according
to
which
Absalom's
hair
weighed
200
shekels
(2
S
14"),
is
probably
to
be
referred
to
this
royal
norm.
Combining
the
evidence
of
the
extant
Bab.
weights
with
the
evidence
of
later
coins
of
various
countries
of
the
ancient
world,
and
with
the
knowledge,
derived
from
a
statement
in
He-rodotus,
that
the
ratio
of
gold
to
silver
was
as
13
J
to
1,
we
obtain
the
following
results:
—
N.
B.
—
One
hea-vy
talent=98-154
lbs.
avoirdupois;
one
heavy
niina=
1
-636
lb.
avoirdupois.
Now
the
pieces
of
A
and
A
of
the
value
of
the
gold
shekel
in
silver
were
the
units
on
which
were
based
systems
known
as
the
Babylonian
or
Persic
and
the
Phoenician
respectively;
the
reason
tor
the
names
being
that
these
two
standards
seem
to
have
been
associated
by
the
Greeks,
the
first
with
Persia,
whose
coins
were
struck
on
this
standard,
the
second
with
the
great
Phoenician
trading
cities,
Sidon,
Tyre,
etc.
For
con-